Learning Objectives

• If Cable Ab Is Subjected To A Tension Of 700 Divided
• If Cable Ab Is Subjected To A Tension Of 700r4
• If Cable Ab Is Subjected To A Tension Of 700
• If Cable Ab Is Subjected To A Tension Of 7000

By the end of this section, you will be able to:

• Define normal and tension forces.
• Apply Newton’s laws of motion to solve problems involving a variety of forces.
• Use trigonometric identities to resolve weight into components.

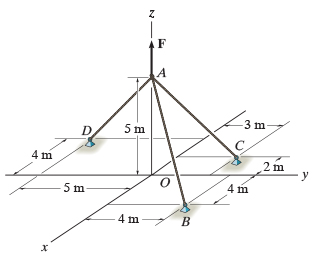
= 700 x 10-6 = 700 m/m = 0.07% Uniaxial Stress and Strain for a prismatic bar, the loads act through the centroid of the cross sections, and the material be homogeneous, the resulting state of stress and strain is called uniaxial stress and strain. If the tension in the cable is 400 N, determine the magnitude and direction of the resultant force acting on the pulley. This angle defines the same angle T of line AB on the tailboard block. Tension in cable BC. Free-Body Diagram: (a) Reaction at A SF A x = =0: 0 x + SM B = + + + 0 75 700 100 550 175 350. BC = +700 N F BC = 700 N. If cable AB is subjected to a tension of 700 N, determine the tension in cables AC and AD and the magnitude of the vertical force F. Please show fully detailed work, have a test coming up and need to know how to do it, not just the answer.

Forces are given many names, such as push, pull, thrust, lift, weight, friction, and tension. Traditionally, forces have been grouped into several categories and given names relating to their source, how they are transmitted, or their effects. The most important of these categories are discussed in this section, together with some interesting applications. Further examples of forces are discussed later in this text.

Weight (also called force of gravity) is a pervasive force that acts at all times and must be counteracted to keep an object from falling. You definitely notice that you must support the weight of a heavy object by pushing up on it when you hold it stationary, as illustrated in Figure 1(a). But how do inanimate objects like a table support the weight of a mass placed on them, such as shown in Figure 1(b)? When the bag of dog food is placed on the table, the table actually sags slightly under the load. This would be noticeable if the load were placed on a card table, but even rigid objects deform when a force is applied to them. Unless the object is deformed beyond its limit, it will exert a restoring force much like a deformed spring (or trampoline or diving board). The greater the deformation, the greater the restoring force. So when the load is placed on the table, the table sags until the restoring force becomes as large as the weight of the load. At this point the net external force on the load is zero. That is the situation when the load is stationary on the table. The table sags quickly, and the sag is slight so we do not notice it. But it is similar to the sagging of a trampoline when you climb onto it.

Figure 1. (a) The person holding the bag of dog food must supply an upward force F_hand equal in magnitude and opposite in direction to the weight of the food w. (b) The card table sags when the dog food is placed on it, much like a stiff trampoline. Elastic restoring forces in the table grow as it sags until they supply a force N equal in magnitude and opposite in direction to the weight of the load.

We must conclude that whatever supports a load, be it animate or not, must supply an upward force equal to the weight of the load, as we assumed in a few of the previous examples. If the force supporting a load is perpendicular to the surface of contact between the load and its support, this force is defined to be a normal force and here is given the symbol N. (This is not the unit for force N.) The word normal means perpendicular to a surface. The normal force can be less than the object’s weight if the object is on an incline, as you will see in the next example.

Common Misconception: Normal Force (N) vs. Newton (N)

Example 1. Weight on an Incline, a Two-Dimensional Problem

Consider the skier on a slope shown in Figure 2. Her mass including equipment is 60.0 kg. (a) What is her acceleration if friction is negligible? (b) What is her acceleration if friction is known to be 45.0 N?

Figure 2. Since motion and friction are parallel to the slope, it is most convenient to project all forces onto a coordinate system where one axis is parallel to the slope and the other is perpendicular (axes shown to left of skier). N is perpendicular to the slope and f is parallel to the slope, but w has components along both axes, namely w_⊥ and [latex]textbf{w}_{parallel}[/latex]. N is equal in magnitude to w_⊥, so that there is no motion perpendicular to the slope, but f is less than w∥, so that there is a downslope acceleration (along the parallel axis).

Strategy

This is a two-dimensional problem, since the forces on the skier (the system of interest) are not parallel. The approach we have used in two-dimensional kinematics also works very well here. Choose a convenient coordinate system and project the vectors onto its axes, creating two connected one-dimensional problems to solve. The most convenient coordinate system for motion on an incline is one that has one coordinate parallel to the slope and one perpendicular to the slope. (Remember that motions along mutually perpendicular axes are independent.) We use the symbols ⊥ and ∥ to represent perpendicular and parallel, respectively. This choice of axes simplifies this type of problem, because there is no motion perpendicular to the slope and because friction is always parallel to the surface between two objects. The only external forces acting on the system are the skier’s weight, friction, and the support of the slope, respectively labeled w, f, and N in Figure 2. N is always perpendicular to the slope, and f is parallel to it. But w is not in the direction of either axis, and so the first step we take is to project it into components along the chosen axes, defining w_∥ to be the component of weight parallel to the slope and w_⊥ the component of weight perpendicular to the slope. Once this is done, we can consider the two separate problems of forces parallel to the slope and forces perpendicular to the slope.

Solution

The magnitude of the component of the weight parallel to the slope is [latex]{w}_{parallel }={w} sin({ 25}^{circ}) = mgsin ({ 25}^{circ})[/latex], and the magnitude of the component of the weight perpendicular to the slope is [latex]{w}_{perp}={w}cos({25}^{circ}) = mgcos({25}^{circ})[/latex].

(a) Neglecting friction. Since the acceleration is parallel to the slope, we need only consider forces parallel to the slope. (Forces perpendicular to the slope add to zero, since there is no acceleration in that direction.) The forces parallel to the slope are the amount of the skier’s weight parallel to the slope w_∥ and friction f. Using Newton’s second law, with subscripts to denote quantities parallel to the slope,

[latex]{a}_{parallel }=frac{{F}_{text{net}parallel }}{m}[/latex]

where [latex]{F}_{text{net}parallel}={w}_{parallel}=mgsin({25^{circ}})[/latex], assuming no friction for this part, so that

is the acceleration.

(b) Including friction. We now have a given value for friction, and we know its direction is parallel to the slope and it opposes motion between surfaces in contact. So the net external force is now

[latex]{F}_{text{net}parallel }={w}_{parallel }-f[/latex]

and substituting this into Newton’s second law, [latex]{a}_{parallel}=frac{{F}_{text{net}parallel }}{m}[/latex], gives

[latex]{a}_{parallel}=frac{{F}_{text{net}parallel}}{m}=frac{{w}_{parallel}-f}{m}=frac{{mg}sin({25}^{circ})-f}{m}[/latex].

We substitute known values to obtain

[latex]{a}_{parallel }=frac{(60.0text{ kg})(9.80text{ m/s}^{2})(0.4226)-45.0text{ N}}{60.0text{ kg}}[/latex]

which yields

[latex]a_{parallel}= 3.39text{ m/s}^{2}[/latex]

which is the acceleration parallel to the incline when there is 45.0 N of opposing friction.

Discussion

Since friction always opposes motion between surfaces, the acceleration is smaller when there is friction than when there is none. In fact, it is a general result that if friction on an incline is negligible, then the acceleration down the incline is a = g sin θ, regardless of mass. This is related to the previously discussed fact that all objects fall with the same acceleration in the absence of air resistance. Similarly, all objects, regardless of mass, slide down a frictionless incline with the same acceleration (if the angle is the same).

Resolving Weight into Components

Figure 3. An object rests on an incline that makes an angle θ with the horizontal.

When an object rests on an incline that makes an angle θ with the horizontal, the force of gravity acting on the object is divided into two components: a force acting perpendicular to the plane, w_⊥, and a force acting parallel to the plane,[latex]textbf{w}_{parallel}[/latex]. The perpendicular force of weight, w_⊥, is typically equal in magnitude and opposite in direction to the normal force, N. The force acting parallel to the plane, [latex]textbf{w}_{parallel}[/latex], causes the object to accelerate down the incline. The force of friction, f, opposes the motion of the object, so it acts upward along the plane. It is important to be careful when resolving the weight of the object into components. If the angle of the incline is at an angle θ to the horizontal, then the magnitudes of the weight components are

[latex]w_{parallel}=w sin{theta} = mg sin{theta}[/latex]

and

[latex]w_{perp}=w cos{theta} = mg cos{theta}[/latex]

Instead of memorizing these equations, it is helpful to be able to determine them from reason. To do this, draw the right triangle formed by the three weight vectors. Notice that the angle θ of the incline is the same as the angle formed between w and w_⊥. Knowing this property, you can use trigonometry to determine the magnitude of the weight components:

[latex]cos({theta})=frac{{w}_{perp}}{w}[/latex]

[latex]w_{perp} = w cos{theta} = mg cos({theta})[/latex]

[latex]sin{(theta)}=frac{{w}_{parallel}}{w}[/latex]

[latex]w_{parallel} = w sin{theta} = mg sin({theta})[/latex]

If Cable Ab Is Subjected To A Tension Of 700 Divided

Take-Home Experiment: Force Parallel

If cable AB is subjected to a tension of 700 N, determine the tension in cables AC and AD and the magnitude of the vertical force F .

If Cable Ab Is Subjected To A Tension Of 700r4

MODULE‐I (16 Lectures) Classification of Engineering Materials, Engineering properties of materials. Characteristic property of metals, bonding in solids, primary bonds like ionic, covalent and metallic bond, crystal systems, common crystal structure of metals, representations of planes and directions in crystals, atomic packing in crystals, calculation of packing...

If Cable Ab Is Subjected To A Tension Of 700

If Cable Ab Is Subjected To A Tension Of 7000